Multiplying fractions is a fundamental skill in maths, pivotal for understanding and solving complex problems in various fields.
This guide aims to simplify the process.
Whether it's for academic purposes or practical applications in daily life, mastering fraction multiplication can significantly enhance your mathematical skills.

Understanding the basics of fractions
Fractions represent parts of a whole, comprising a numerator (top number) and a denominator (bottom number).
Grasping the different types of fractions—proper, improper, and mixed numbers—is crucial.
Proper fractions have a numerator smaller than the denominator.
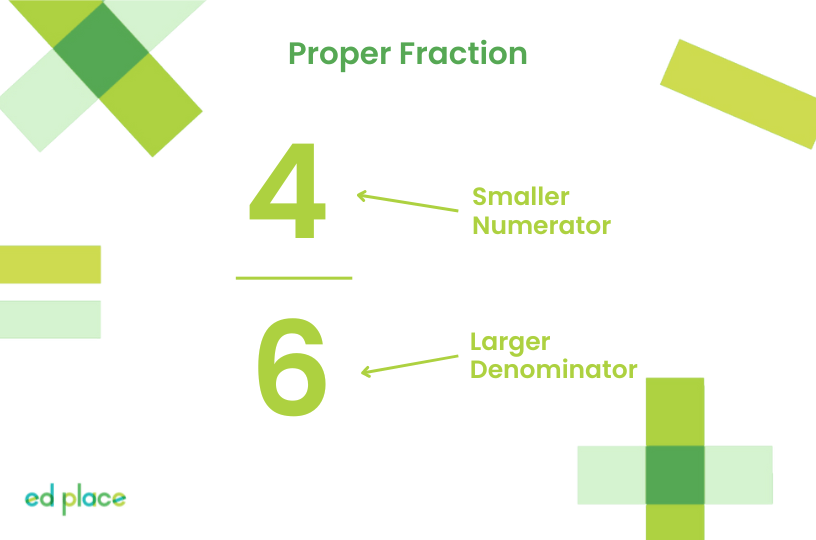
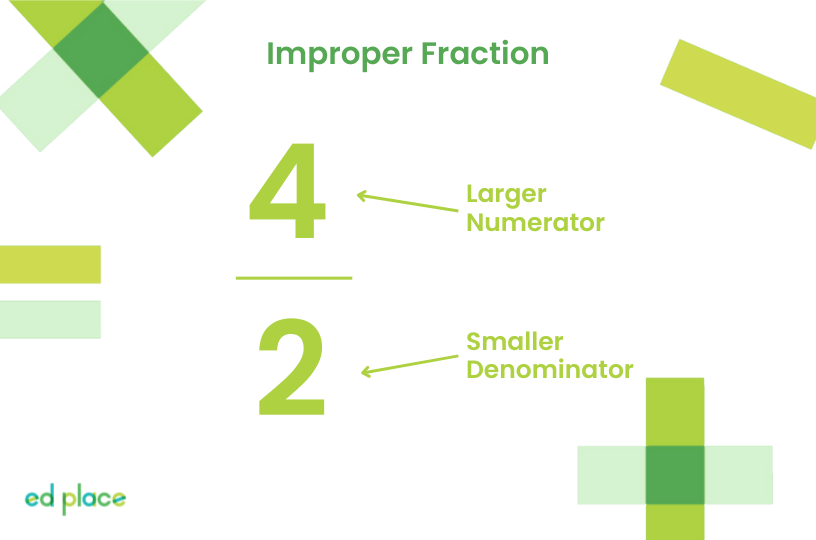
Improper fractions have a larger numerator.
Mixed numbers combine whole numbers with fractions.
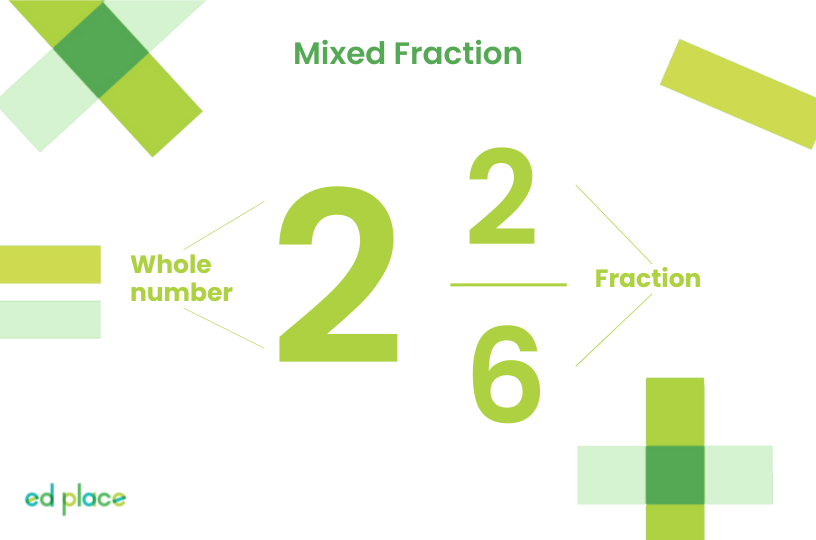
The process of multiplying fractions
Multiplying fractions is straightforward: multiply the numerators together and then the denominators.
Unlike addition or subtraction, you don't need to find a common denominator.
Simplifying the result to its lowest terms ensures the answer is in its simplest form, making it easier to understand and use in further calculations.
For example:
Multiply the fractions 1/2 and 3/4.
First, you multiply the numerators (1 x 3 = 3).
Then multiply the denominators (2 x 4 = 8).
This leaves us with the fraction 3/8.
This fraction is already in its simplest form, demonstrating the importance of simplifying to make fractions easier to understand and use.
Simplification before and after multiplication
Simplification before and after multiplying fractions is a crucial step to streamline the calculation process and ensure the results are presented in the simplest form.
Before multiplication, check if the fractions can be simplified by dividing the numerator and denominator by their greatest common divisor. This preemptive simplification can make the multiplication step easier and sometimes more straightforward.
For instance:
If you multiply 2/4 by 3/9, you can simplify each fraction first.
2/4 simplifies to 1/2 (since 2 is a common factor of both the numerator and denominator).
3/9 simplifies to 1/3 (as 3 is common to both).
Then, multiply the simplified fractions: 1/2 × 1/3 = 1/6.
This approach streamlines the multiplication process and ensures the final answer is in its simplest form right from the start.
Multiplying fractions with whole numbers
Multiplying a fraction by a whole number involves a simple conversion: representing the whole number as a fraction with a denominator of one.
This transforms the multiplication process into a familiar fraction-by-fraction operation, reducing it down to normal fraction multiplication rules. The product, often an improper fraction, can then be converted into a mixed number if needed.
Example:
If you multiply the fraction 2/3 by the whole number 4.
First express the whole number as a fraction (4/1).
Then, multiply the numerators (2 x 4 = 8).
Now, multiply the denominators (3 x 1 = 3).
This results in 8/3.
Since 8/3 is an improper fraction, it can be converted to 2 2/3.
![]() Challenge: Now, try multiplying the fraction 5/6 by the whole number 3. Convert your answer to its simplest form.
Challenge: Now, try multiplying the fraction 5/6 by the whole number 3. Convert your answer to its simplest form.
Answer:
To solve the challenge, multiply the fraction 5/6 by the whole number 3.
First, convert 3 to a fraction (3/1), making the multiplication 5/6 × 3/1.
Multiplying the numerators gives 15, and multiplying the denominators gives 6, resulting in 15/6.
Simplifying this fraction to its lowest terms, you get 2 1/2.
Would you like to explore this further?
Take on our online worksheet Multiplying Proper Fractions by Whole Numbers.
Multiplying mixed numbers
To multiply mixed numbers, first convert them into improper fractions. Check out our worksheet if you need a refresher on this.
This unifies the format of the numbers, leading to more straightforward multiplication. The process underscores the importance of versatility in handling fractions, ensuring learners can manage various fractional forms effectively. After multiplication, the product might require conversion back into a mixed number.
Example:
Multiply the mixed numbers 2 1/4 and 3 1/3.
First convert them into improper fractions.
2 1/4 becomes 9/4 and 3 1/3 becomes 10/3.
Multiply these improper fractions: 9/4×10/3=90/12.
Simplify to 7 1/2.
Remember, practice makes perfect. With each fraction you simplify, you're sharpening your skills for real-world challenges.
For more practise check out our worksheet, Multiply Fractions Including Mixed Numbers.
Practice problems and solutions
To cement your understanding of multiplying fractions, here are some practice problems along with their solutions for self-assessment:
1) Multiply 1/3 by 4/5.
2) Multiply and simplify 2/8 by 3/6.
3) Multiply 5/6 by 2/3 and simplify.
4) Multiply 7/9 by 3/4.
5) Multiply 3/7 by 5/8 and simplify to its lowest terms.
Answers:
1) Multiplying the numerators (1×4) and denominators (3×5) results in 4/15.
2) Simplifying each fraction to 1/4 and 1/2 respectively. Then multiplying gives 1/8.
3) Multiplying the numerators (5×2) and denominators (6×3) gives 10/18. Simplifying this fraction results in 5/9.
4) Multiplying 7/9 by 3/4 results in 21/36. Simplifying this fraction gives 7/12.
5) The product of 3/7 and 5/8 is 15/56, which is already in its simplest form.
In this guide, we've covered the foundational aspects of multiplying fractions, including the steps for multiplication, the importance of simplifying fractions, and strategies for handling whole numbers and mixed numbers.
Regular practice of these principles can significantly improve your confidence and proficiency in dealing with fractions. Encourage yourself to tackle a variety of problems, and remember, mastering fraction multiplication opens up new avenues in mathematical understanding and application.
Use our worksheets below to keep practicing, and soon, multiplying fractions will become second nature.







.png)
.png)
.png)




