A quadrilateral, at its simplest, is any shape that has four straight sides.
But why stop there?
Each side, each angle, and the way they connect gives us an idea of the different type of quadrilateral it is—whether it's a square, a rectangle, a kite, or one of the other interesting types we'll explore. Quadrilaterals are everywhere: from the frame of your classroom window to the screen of your smartphone, they form the building blocks of many objects you interact with every day. Just take a look around and you’ll see them everywhere!
Importance of Learning About Quadrilaterals
Let's take a closer look at the various types of quadrilaterals. Each one has its own unique set of properties, which we’ll summarise below. This will help you recognise and tell the difference between them easily!
| Name | Shape | Main Features |
|---|---|---|
| Square |
|
- All sides equal - All angles are 90° |
| Rectangle |  |
- Opposite sides are equal - All angles are 90° |
| Trapezium | 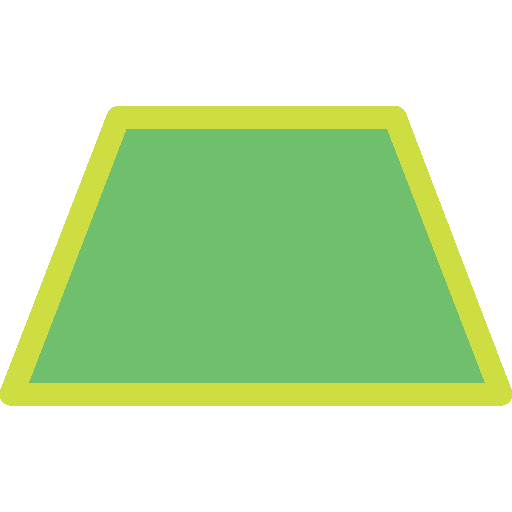 |
- At least one pair of parallel sides |
| Rhombus |  |
- All sides are equal - Opposite angles are equal |
| Parallelogram | 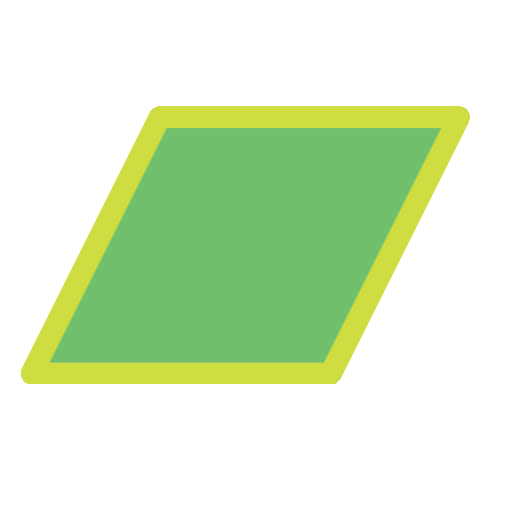 |
- Opposite sides are equal and parallel - Opposite angles are equal |
| Kite |  |
- Two pairs of adjacent sides are equal - One pair of opposite angles are equal |
Properties of a Square
A square is perhaps the most well-known and easily recognisable of all quadrilaterals. It's a regular polygon which is quickly identified because of its symmetry and simplicity.

Defining Features of a Square
- Sides: All four sides of a square are exactly the same length, making it perfectly symmetrical.
- Angles: Each of the four angles in a square is a right angle, which measures 90 degrees. This uniformity helps in creating a balanced and straightforward shape.
How to Identify a Square
Recognising a square is quite straightforward, but here are some tips to ensure you're spot on:
- Equal Sides: Check if all sides are of the same length. This is the first and most crucial step.
- Right Angles: Use a right-angle finder or a simple square tool to check each corner. If all angles are right angles, you're looking at a square.
- Symmetry: Draw a line through the square from any corner to the opposite corner (diagonally). If this line divides the square into two equal right triangles, then it confirms the shape is a square.
Squares are everywhere! From the tiles on your floor to classic board games, they form an essential part of the designs and patterns we see and use every day. By understanding these basic properties, you can easily spot and utilise squares in both academic problems and daily observations.
Properties of a Rectangle
A rectangle, like the square, is among the most common and easily identifiable quadrilaterals. It's distinguished primarily by its longer sides and right angles.
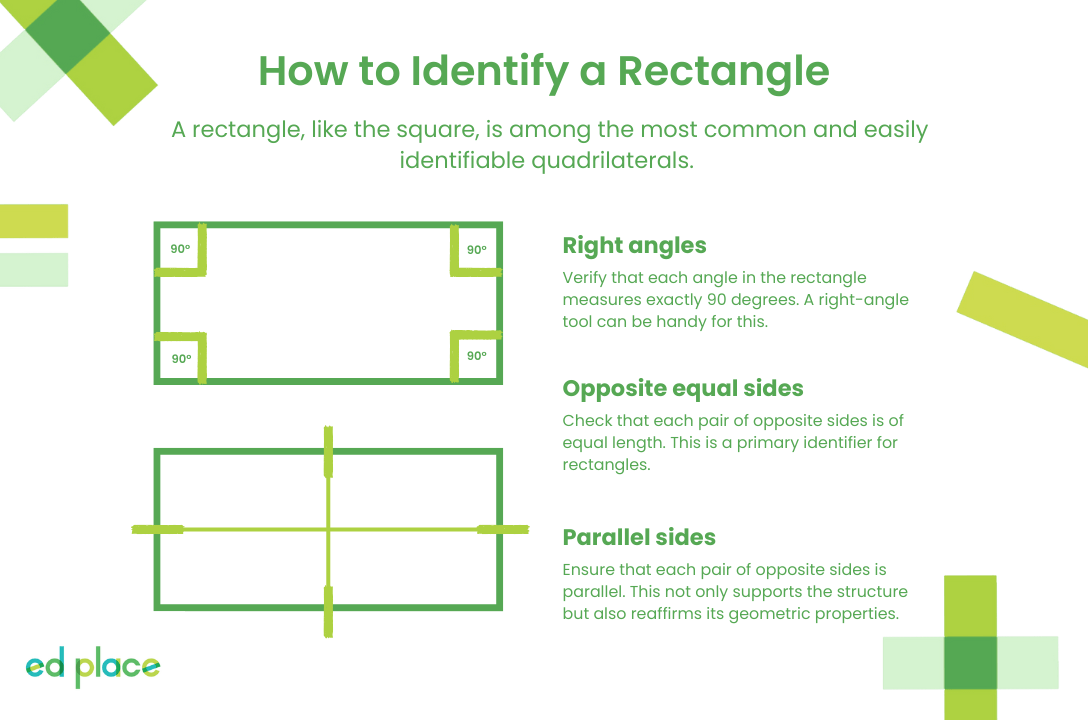
Key Characteristics of a Rectangle
- Sides: Rectangles have opposite sides that are equal in length. This means the two longer sides are the same length, as are the two shorter sides, forming a pair each.
- Angles: All four corners of a rectangle are right angles (90 degrees). This makes rectangles a cornerstone in the world of geometry due to their predictability and ease of calculation.
How to Identify a Rectangle
Identifying a rectangle involves a few simple observations:
- Opposite Equal Sides: Check that each pair of opposite sides is of equal length. This is a primary identifier for rectangles.
- Right Angles: Verify that each angle in the rectangle measures exactly 90 degrees. A right-angle tool can be handy for this.
- Parallel Sides: Ensure that each pair of opposite sides is parallel. This not only supports the structure but also reaffirms its geometric properties.
Rectangles are integral to many aspects of both design and everyday functionality. From windows and doors to books and mobile devices, their presence is ubiquitous. Knowing how to spot and describe rectangles can enhance both your geometric understanding and your appreciation of the world around you.
Properties of a Trapezium
A trapezium is a four-sided figure that stands out with its unique shape, setting itself apart from the perfect symmetry of squares and rectangles. It's the quirky character of the quadrilateral family! A trapezium’s defining feature is having at least one pair of parallel sides, which sets it apart in the world of quadrilaterals.
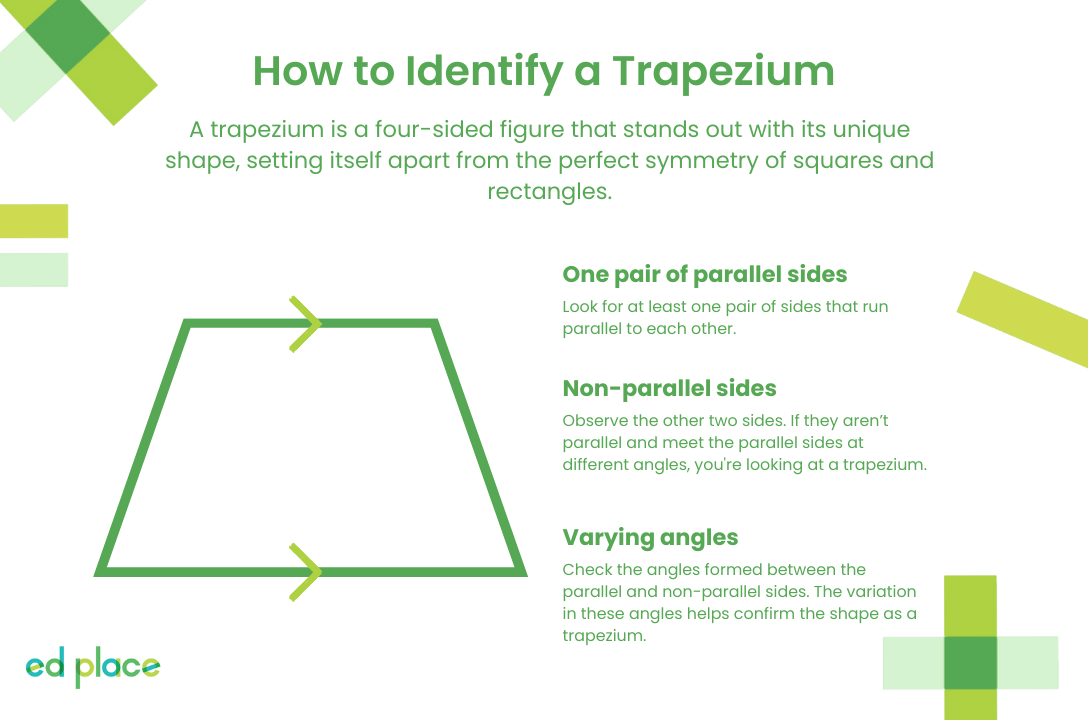
Key Characteristics of a Trapezium
- Sides: A trapezium has one pair of opposite sides that are parallel, known as the bases. The other two sides, which are not parallel, are referred to as the legs.
- Angles: The angles adjacent to each base can be different. This means that the angles on one side of a trapezium can be acute and the other side obtuse, depending on the length of the sides and the shape of the trapezium.
An acute angle is an angle that measures less than 90 degrees. An obtuse angle is an angle that measures more than 90 degrees, and less than 180 degrees.
How to Identify a Trapezium
Identifying a trapezium involves checking for its unique side and angle arrangements:
- One Pair of Parallel Sides: Look for at least one pair of sides that run parallel to each other. This is the most crucial feature distinguishing a trapezium from other quadrilaterals.
- Non-parallel Sides: Observe the other two sides. If they aren’t parallel and meet the parallel sides at different angles, you're looking at a trapezium.
- Varying Angles: Check the angles formed between the parallel and non-parallel sides. The variation in these angles helps confirm the shape as a trapezium.
Trapeziums are frequently used in architecture and design, providing interesting visual elements due to their occasional lack of symmetry. Recognising these shapes in your surroundings can enhance your understanding of different quadrilaterals and their applications.
Properties of a Rhombus
A rhombus is often likened to a diamond because of its equally angled sides and overall symmetry. This quadrilateral is unique in that all of its sides are of equal length, but unlike a square, its different angles can vary.
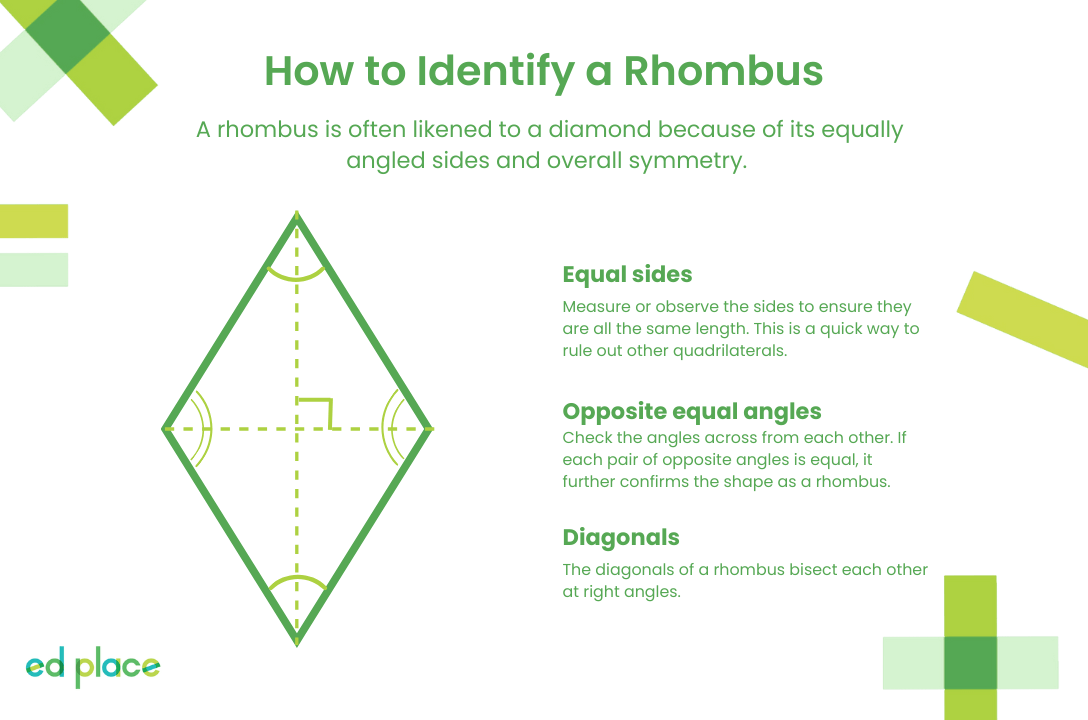
Key Characteristics of a Rhombus
- Sides: All four sides of a rhombus are of equal length, just like a square.
- Angles: The opposite angles of a rhombus are equal. Typically, one pair of opposite angles is acute, and the other pair is obtuse, creating a somewhat tilted appearance.
How to Identify a Rhombus
To determine if a shape is a rhombus, you can use the following guidelines:
- Equal Sides: Measure or observe the sides to ensure they are all the same length. This is a quick way to rule out other quadrilaterals.
- Opposite Equal Angles: Check the angles across from each other. If each pair of opposite angles is equal, it further confirms the shape as a rhombus.
- Diagonals: The diagonals of a rhombus bisect each other at right angles. This property is not only unique but also useful in confirming a rhombus if the side lengths and angles are consistent with the shape’s definitions.
Rhombuses are found in various forms of art and design, especially in tiling patterns and jewellery designs. Understanding how to identify and utilise rhombuses can later be a valuable skill in both academic and practical applications.
Properties of a Parallelogram
The parallelogram is a cool quadrilateral that looks like a slanted rectangle. It has pairs of opposite sides that are not only equal in length but also run perfectly parallel to each other, giving it a unique tilted look.
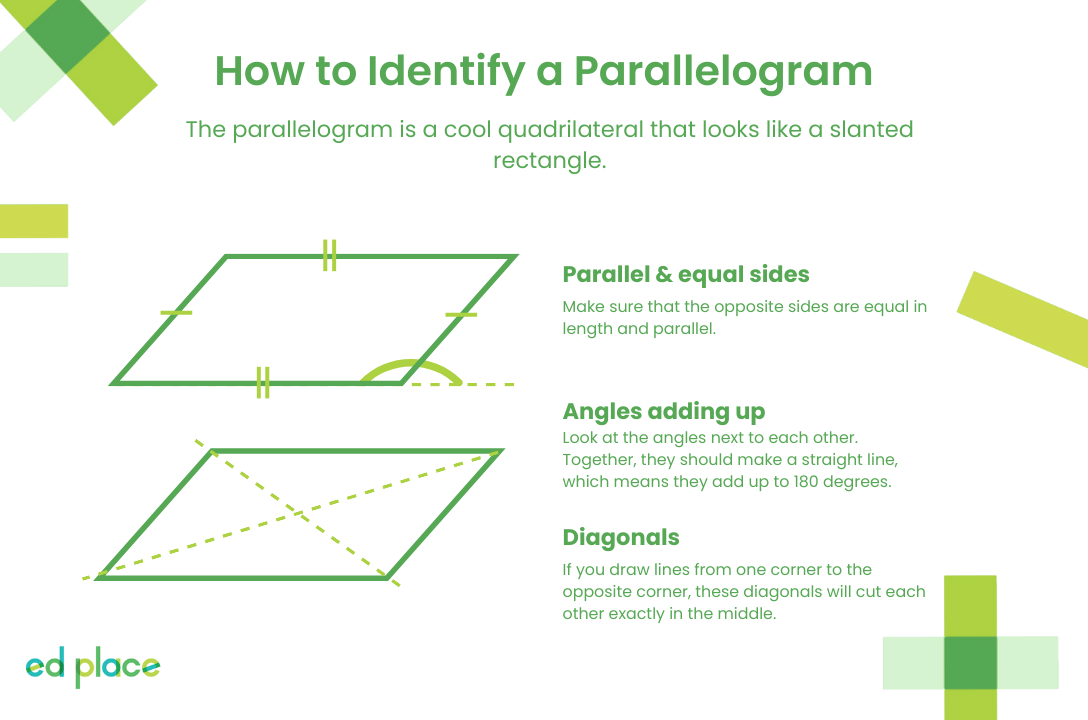
Key Characteristics of a Parallelogram
- Sides: In a parallelogram, each pair of opposite sides is the same length and runs straight alongside each other without meeting.
- Angles: The angles across from each other in a parallelogram are the same. The angles next to each other (we call these adjacent angles) always add up to 180 degrees. This means if one angle is 60 degrees, the one next to it has to be 120 degrees to make the total 180 degrees.
How to Identify a Parallelogram
Looking for a parallelogram? Here's what you should check:
- Parallel and Equal Sides: Make sure that the opposite sides are equal in length and parallel. This is the most important feature of a parallelogram.
- Angles Adding Up: Look at the angles next to each other. Together, they should make a straight line, which means they add up to 180 degrees.
- Diagonals: If you draw lines from one corner to the opposite corner, these diagonals will cut each other exactly in the middle. This is another cool trick to identify a parallelogram.
Parallelograms are everywhere! From the way bridges are built to some of the cool art you might see in a museum, understanding how to spot and understand parallelograms can make you see things a little differently. They show how geometry isn't just about boring rules but about making things work and look good in the real world too.
Properties of a Kite
The kite is a playful quadrilateral that resembles the flying toy it's named after. It's easy to spot thanks to its unique look, where two pairs of adjacent sides are of equal length but the overall shape isn't symmetrical like a square or rectangle.
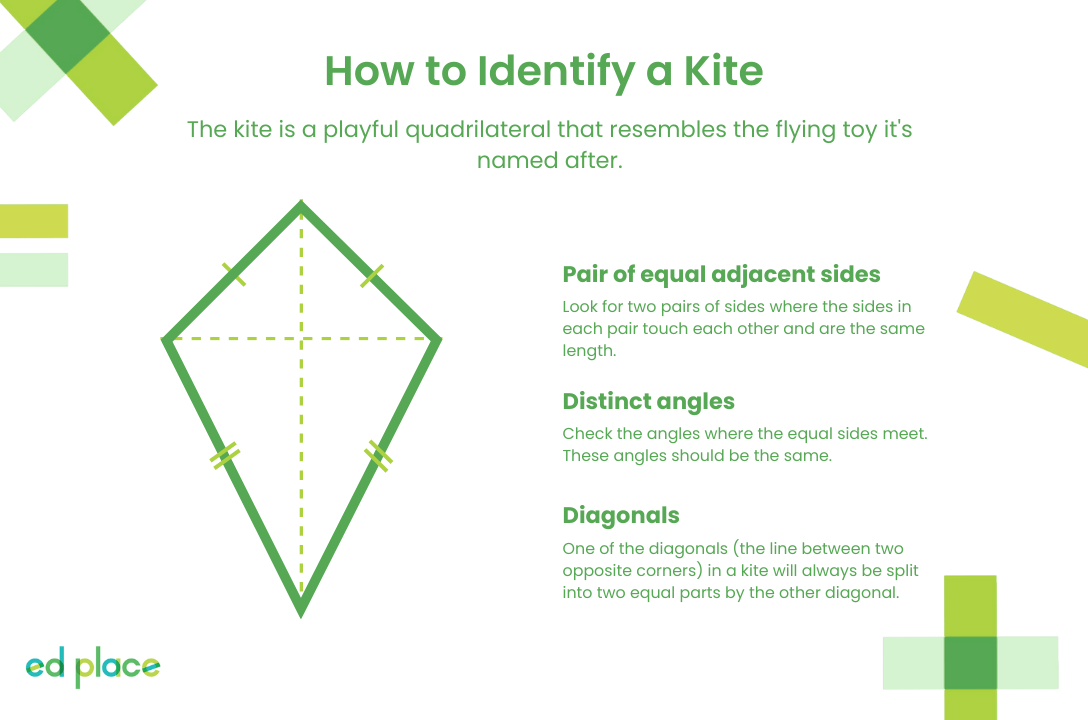
Key Characteristics of a Kite
- Sides: A kite has two pairs of sides that are next to each other and are the same length. Imagine drawing a kite: you start with two sticks of one length crossed by two sticks of another length.
- Angles: The angles where the sides of the same length meet are always equal. These are typically the angles at the top and bottom of the kite, where the shorter side meets the longer side.
How to Identify a Kite
Here’s how you can tell if a shape is a kite:
- Pair of Equal Adjacent Sides: Look for two pairs of sides where the sides in each pair touch each other and are the same length. This is the most telling feature of a kite.
- Distinct Angles: Check the angles where the equal sides meet. These angles should be the same. It helps to think of the kite you might fly—where the string attaches usually forms a sharper angle.
- Diagonals: One of the diagonals (the line between two opposite corners) in a kite will always be split into two equal parts by the other diagonal. This isn't something you might easily see without drawing it out, but it’s a neat property if you’re working with paper and pencil.
Kites are not just fun to fly; they’re also interesting to study in geometry. You might see the kite shape in various designs and patterns, especially in artwork and quilts. Understanding kites helps you appreciate the diversity and creativity in geometry, showing how different shapes can be used in fun and practical ways.
Recap Your Knowledge!
Now we’ve looked at the different types of quadrilaterals and their properties, have a go at this quick quiz to see if you remember everything you’ve learnt!
-
Which quadrilateral has opposite sides that are parallel and all angles are right angles?
- A) Kite
- B) Parallelogram
- C) Rectangle
- D) Trapezium
-
What is unique about the sides of a rhombus?
- A) All sides are parallel.
- B) Opposite sides are of unequal lengths.
- C) All sides are of equal length.
- D) No sides are equal.
-
If a quadrilateral has exactly one pair of parallel sides, what shape is it most likely to be?
- A) Square
- B) Trapezium
- C) Rhombus
- D) Kite
-
Which statement is true for both a rhombus and a parallelogram?
- A) All angles are 90 degrees.
- B) Diagonals bisect each other at right angles.
- C) Opposite sides are parallel.
- D) All sides are of the same length.
-
A quadrilateral with two pairs of adjacent equal sides is called what?
- A) Rectangle
- B) Square
- C) Kite
- D) Parallelogram
Answers
- Answer: C) Rectangle
- Answer: C) All sides are of equal length
- Answer: B) Trapezium
- Answer: C) Opposite sides are parallel
- Answer: C) Kite
Check out the following worksheets to try your hand at a few extra challenges, including the next step of finding angles in quadrilaterals!




.png)
.png)
.png)




